NX Nastran Connection Elements (RBE2, RBE3 and CBUSH) and How Amazingly Useful They Are for FEA Modeling
Objective
This white paper assumes that the reader has the basics of FEA down pat and an inkling of how R-elements work. The objective is to describe in detail how to use R-connections and CBUSH elements correctly and with confidence. If you make it through this note, you’ll most likely know more about these little connections than 99% of your peers.
Introduction: We’ll cover the basics of MPC terminology which is the foundation of the RBE2 and RBE3 connections. A keen understanding will be provided on how to think in terms of independent and dependent nodes. It’ll be obvious after this discussion that it is not logical to apply SPC’s to dependent nodes or to connect other dependent nodes between different R-elements. Best practices will be covered and some recommendations given (e.g., be careful when using MPC’s in a nonlinear analysis and especially so when large displacements are involved).
The thermal CTE capability of the RBE2 connection will also be covered for completeness.
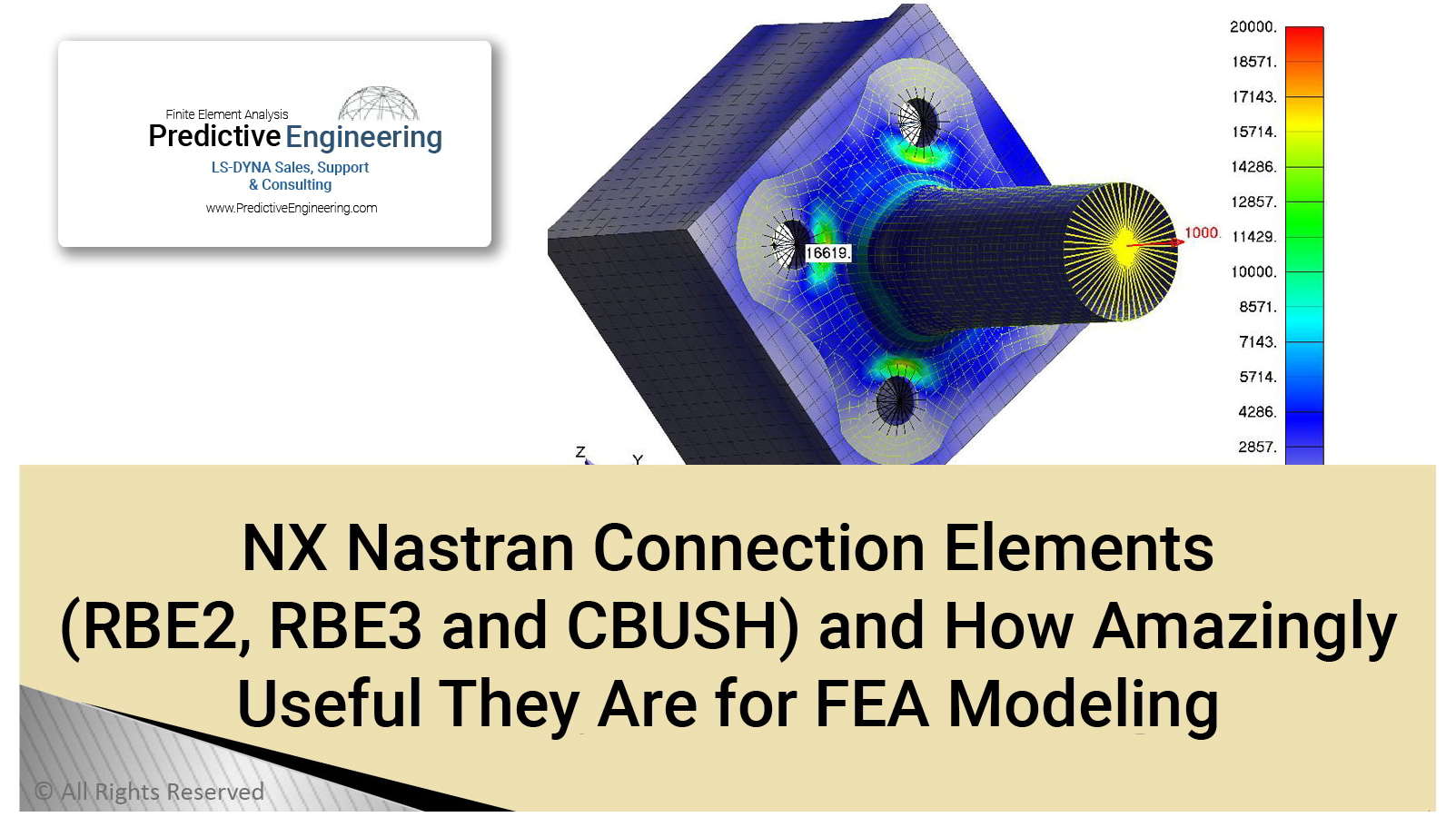
Lastly, the CBUSH element will be introduced and applications given on how to use this replacement for the CELAS element. The downfalls on using this element will be discussed and also why this element is a useful as a companion to the RBE2 and RBE3 elements.
The Basics About Multi-Point Constraints (RBE2 and RBE3 Elements): The tomes that have been written about R-type elements number in the dozens. In their usage they have friends and foes. For one highly experienced Nastran engineer that I know, the use of RBE3 elements is generally prohibited among their crew simply due to their ability to suck the life (i.e., load) out of a standard analysis run and such death-inducing ability is often reason enough to just say “no” to their usage.
To get a handle on how R-elements work, it is best to think of them as multi-point constraints (MPC). Which then leads to the question as to what exactly is a MPC? Taking a look at the NX Nastran Element Library Reference, one is presented with some dense logic but at its core, it defines a MPC as a linear equation among chosen displacements that is equal to zero.
